Toptailieu.vn xin giới thiệu Hai tam giác đồng dạng (Chân trời sáng tạo) hay, chi tiết | Lý thuyết Toán 8. Bài viết gồm phần lý thuyết trọng tâm nhất được trình bày một cách dễ hiểu, dễ nhớ bên cạnh đó là bộ câu hỏi trắc nghiệm có hướng dẫn giải chi tiết để học sinh có thể vận dụng ngay lý thuyết, nắm bài một cách hiệu quả nhất. Mời các bạn đón xem:
Lý thuyết Hai tam giác đồng dạng (Chân trời sáng tạo) hay, chi tiết | Lý thuyết Toán 8
A. Lý thuyết Hai tam giác đồng dạng
1. Khái niệm
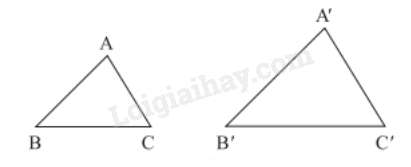
Tam giác A’B’C’ gọi là đồng dạng với tam giác ABC nếu:
và
Kí hiệu: (viết theo thứ tự cặp đỉnh tương ứng).
Tỉ số là tỉ số đồng dạng của
với
.
2. Tính chất
Tính chất 1. Mỗi tam giác đồng dạng với chính nó theo tỉ số k = 1.
Tính chất 2. Nếu theo tỉ số k thì
theo tỉ số
.
Ta nói và
đồng dạng với nhau.
Tính chất 3.
3. Định lí
Nếu một đường thẳng cắt hai cạnh của một tam giác và song song với cạnh còn lại thì nó tạo thành một tam giác mới đồng dạng với tam giác đã cho.
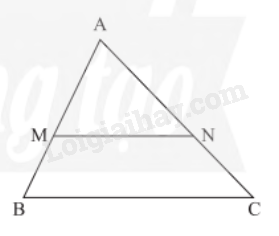
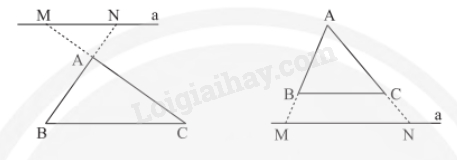
Chú ý: Định lí trên cũng đúng trong trường hợp đường thẳng a cắt phần kéo dài của hai cạnh và song song với cạnh còn lại.
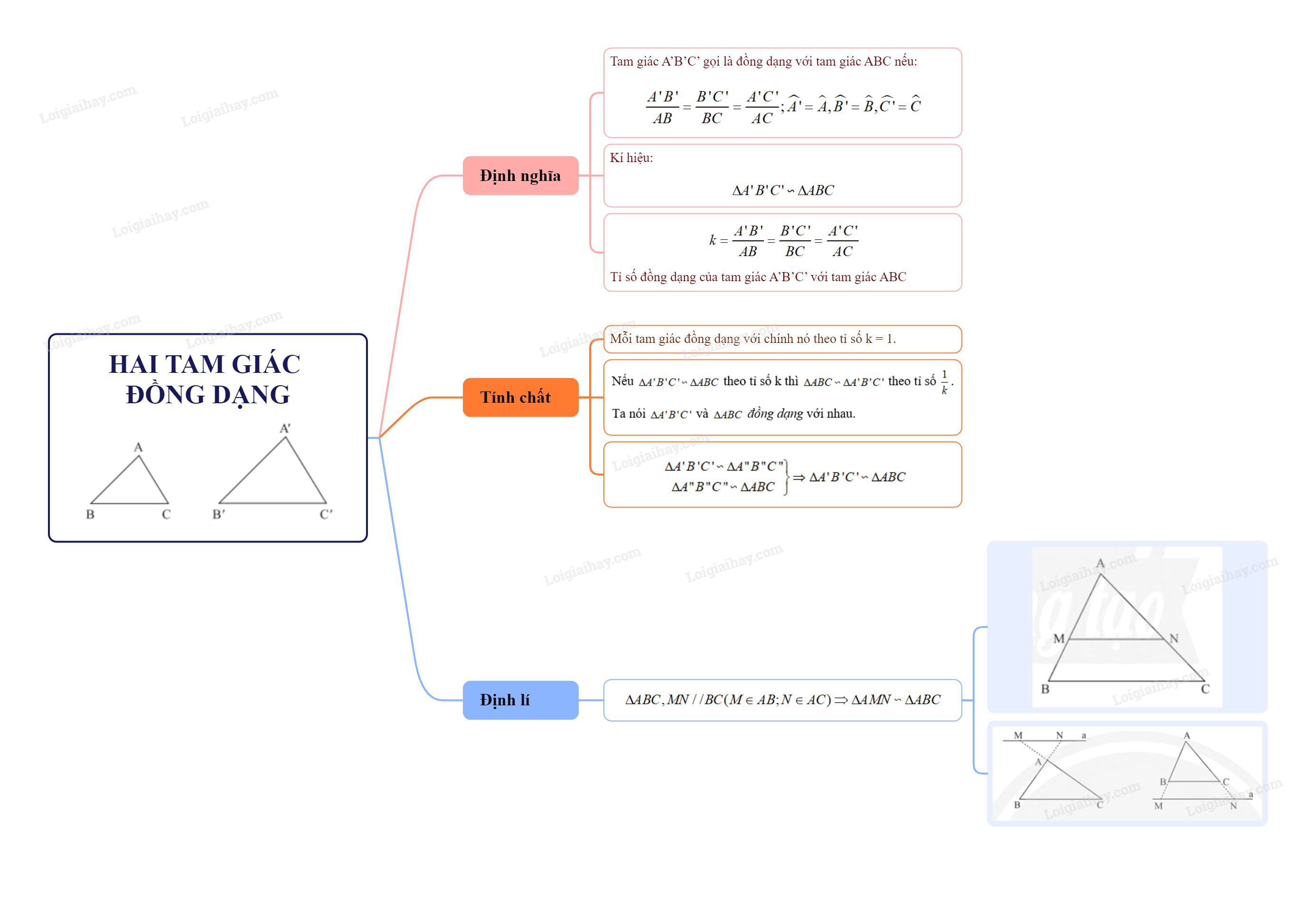
Sơ đồ tư duy Hai tam giác đồng dạng
B. Bài tập Hai tam giác đồng dạng
Đang cập nhật...
Xem thêm các bộ Lý thuyết Toán 8 (Chân trời sáng tạo) hay, chi tiết khác:
CÔNG TY TNHH ĐẦU TƯ VÀ DỊCH VỤ GIÁO DỤC VIETJACK
- Người đại diện: Nguyễn Thanh Tuyền
- Số giấy chứng nhận đăng ký kinh doanh: 0108307822, ngày cấp: 04/06/2018, nơi cấp: Sở Kế hoạch và Đầu tư thành phố Hà Nội.
2021 © All Rights Reserved.