Phương pháp giải Công thức tính điện thế (50 bài tập minh họa)

Bạn cần đăng nhập để báo cáo vi phạm tài liệu

Phương pháp giải Công thức tính điện thế (50 bài tập minh họa)

Ngọc Nguyễn Ngày: 17-07-2023 Lớp 11

321

Toptailieu.vn biên soạn và giới thiệu Phương pháp giải Công thức tính điện thế (50 bài tập minh họa) hay, chi tiết nhất, từ cơ bản đến nâng cao giúp học sinh nắm vững kiến thức, từ đó học tốt môn Vật lí 11.

Nội dung bài viết

1. Định nghĩa
2. Công thức
3. Mở rộng
4. Ví dụ minh họa

Phương pháp giải Công thức tính điện thế (50 bài tập minh họa)

1. Định nghĩa

- Điện thế tại một điểm trong điện trường là đại lượng đặc trưng riêng cho điện trường về phương diện tạo ra thế năng khi đặt tại đó một điện tích q. Nó được xác định bằng thương số giữa công của lực điện tác dụng lên điện tích q khi q di chuyển từ M ra xa vô cùng và độ lớn của q.

2. Công thức

- Điện thế tại điểm M trong điện trường: $V_{M} = \frac{W_{M}}{q} = \frac{A_{M \infty}}{q}$ .

- Trong đó:

+ $V_{M}$ : Điện thế tại điểm M trong điện trường (V)

+ $W_{M}$ : Thế năng của một điện tích q tại điểm M trong điện trường (J)

+ $A_{M \infty}$ : Công của lực điện tác dụng lên điện tích q khi q di chuyển từ M ra xa vô cùng

+ q: điện tích (C)

3. Mở rộng

- Điện thế tại điểm M gây bởi điện tích q: $V_{M} = k \frac{q}{r}$ .

- Nếu các điện tích q₁, q₂, …, q_n gây ra tại điểm M các điện thế V₁, V₂, …, V_n thì điện thế toàn phần gây ra bởi hệ điện tích được tính là: V = V₁ + V₂ + V₃ + ...+ V_n

- Hiệu điện thế V_M – V_N = U_MN = $\frac{A_{M}}{q}$

4. Ví dụ minh họa

Ví dụ 1: Thế năng của một electron tại điểm M trong điện trường của một điện tích điểm là $- 3, 2 . 10^{- 19} J$ . Điện thế tại điểm M là?

Hướng dẫn giải:

+ Áp dụng công thức $V_{M} = \frac{W_{M}}{q} = \frac{- {3,2.10}^{- 19}}{- {1,6.10}^{- 19}} = + 2 (V) .$

Ví dụ 2: Khi di chuyển điện tích q = −10^-4 C từ rất xa (vô cực) đến điểm M trong điện trường thì công của lực điện thực hiện là 5.10^-5 J. Cho điện thế ở vô cực bằng 0. Điện thế ở điểm M là bao nhiêu?

Hướng dẫn giải:

Ta có

$V_{\infty} - V_{M} = U = \frac{A}{q} = \frac{{5.10}^{- 5}}{- 10^{- 4}} = - 0,5 \Rightarrow V_{M} = 0,5 V .$

Ví dụ 3: Có ba điện tích điểm $q_{1} = {15.10}^{- 9} C;$ $q_{2} = - {12.10}^{- 9} C;$ $q_{3} = {7.10}^{- 9} C$ đặt tại 3 đỉnh của tam giác đều ABC, với cạnh 10 cm. Điện thế tại tâm O và điểm H – chân đường cao từ A xuống BC do ba điện tích này gây ra là?

Hướng dẫn giải:

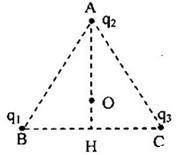
Công thức tính điện thế hay nhất | Cách tính điện thế | Vật lý lớp 11 (ảnh 1)

Điện thế tại O:

$V_{0} = V_{10} + V_{20} + V_{30} = k \frac{q_{1}}{O A} + k \frac{q_{2}}{O B} + k \frac{q_{3}}{O C}$

Ta có, tam giác ABC đều

$\Rightarrow O A = O B = O C = \frac{2}{3} . \frac{10 \sqrt{3}}{2} = \frac{10}{\sqrt{3}} c m = \frac{0,1}{\sqrt{3}} m$

$\Rightarrow V_{0} = \frac{k}{O A} (q_{1} + q_{2} + q_{3}) = \frac{{9.10}^{9}}{\frac{0,1}{\sqrt{3}}} ({15.10}^{- 9} - {12.10}^{- 9} + {7.10}^{- 9}) = 1558,8 V$

- Điện thế tại H do các điện tích điểm gây ra là:

$V_{H} = V_{1 H} + V_{2 H} + V_{3 H} = k \frac{q_{1}}{A H} + k \frac{q_{2}}{B H} + k \frac{q_{3}}{C H}$

Ta có $\{\begin{cases} A H = \frac{10 \sqrt{3}}{2} c m = 0,05 \sqrt{3} m \\ H B = H C = 5 c m = 0,05 m \end{cases}$

$\Rightarrow V_{H} = {9.10}^{9} (\frac{{15.10}^{- 9}}{0,05 \sqrt{3}} + \frac{- {12.10}^{- 9}}{0,05} + \frac{{7.10}^{- 9}}{0,05}) = 658,8 V$

Xem thêm các dạng Vật lí 11 hay, chọn lọc khác:

Công thức tính thế năng của điện tích hay nhất | Cách tính thế năng của điện tích

Công thức tính hiệu điện thế hay nhất | Cách tính hiệu điện thế

Công thức tính tụ điện hay nhất | Cách tính tụ điện

Công thức tính tụ điện mắc nối tiếp hay nhất | Cách tính tụ điện mắc nối tiếp

Công thức tính tụ điện mắc song song hay nhất | Cách tính tụ điện mắc song song