SBT Toán 7 Kết nối tri thức Bài 28: Phép chia đa thức một biến

Giang Ngày: 01-02-2023 Lớp 7

1.1 K

Toptailieu biên soạn và giới thiệu giải sách bài tập Toán 7 Bài 28: Phép chia đa thức một biến sách Kết nối tri thức hay nhất, chi tiết sẽ giúp học sinh dễ dàng làm các bài tập từ đó nâng cao kiến thức và biết cách vận dụng phương pháp giải vào các bài tập trong SBT Toán 7 Bài 28.

Giải SBT Toán 7 Bài 28 (Kết nối tri thức): Phép chia đa thức một biến

Bài 7.25 trang 34 sách bài tập Toán 7: Tìm số tự nhiên n sao cho đa thức 1,2x⁵ - 3x⁴ + 3,7x² chia hết cho xⁿ.

Phương pháp giải

Đa thức đã cho chia hết cho $x^{n}$ nếu từng hạng tử của nó chia hết cho $x^{n}$ nếu từng hạng tử của nó chia hết cho $x^{n}$ .

Lời giải

Đa thức đã cho chia hết cho $x^{n}$ nếu từng hạng tử của nó chia hết cho $x^{n}$ nếu từng hạng tử của nó chia hết cho $x^{n}$ .

$\Rightarrow {\begin{cases} x^{5} ⋮ x^{n} \\ x^{4} ⋮ x^{n} \\ x^{2} ⋮ x^{n} \end{cases} \Rightarrow {\begin{cases} n \leq 5 \\ n \leq 4 \\ n \leq 2 \end{cases} \Rightarrow n \leq 2 \Rightarrow n \in {0; 1; 2}$

Bài 7.26 trang 34 sách bài tập Toán 7: Thực hiện các phép chia sau: a)(- 4x⁵ + 3x³ - 2x²) : (-2x²)

$a) (- 4 x^{5} + 3 x^{3} - 2 x^{2}) : (- 2 x^{2})$

$b) (0, 5 x^{3} - 1, 5 x^{2} + x) : 0, 5 x;$

$c) (x^{3} + 2 x^{2} - 3 x + 1) : \frac{1}{3} x^{2}$ .

Phương pháp giải

Chia từng hạng tử của đa thức bị chia cho đa thức chia rồi tính tổng các thương vừa thu được

Lời giải

$\begin{array}{l} a) \\ (- 4 x^{5} + 3 x^{3} - 2 x^{2}) : (- 2 x^{2}) \\ = (- 4 x^{5}) : (- 2 x^{2}) + 3 x^{3} : (- 2 x^{2}) + (- 2 x^{2}) : (- 2 x^{2}) \\ = 2 x^{3} - \frac{3}{2} x + 1 \end{array}$

$\begin{array}{l} b) \\ (0, 5 x^{3} - 1, 5 x^{2} + x) : 0, 5 x \\ = (0, 5 x^{3} : 0.5 x) - (1, 5 x^{2} : 0, 5 x) + (x : 0, 5 x) \\ = x^{2} - 3 x + 2 \end{array}$

$\begin{array}{l} c) \\ (x^{3} + 2 x^{2} - 3 x + 1) : \frac{1}{3} x^{2} \\ = (3 x + 6) . \frac{1}{3} x^{2} + (- 3 x + 1) \end{array}$

Do đa thức -3x + 1 có bậc là 1, nhỏ hơn bậc 2 của đa thức chia nên đẳng thức này chứng tỏ 3x + 6 là thương và -3x + 1 là dư trong phép chia đã cho.

Bài 7.27 trang 34 sách bài tập Toán 7: Đặt tính và làm phép chia sau: a) (x³ - 4x² - x +12) : (x-3)

$a) (x^{3} - 4 x^{2} - x + 12) : (x - 3)$

$b) (2 x^{4} - 3 x^{3} + 3 x^{2} + 6 x - 14) : (x^{2} - 2)$ .

Phương pháp giải

Đặt phép tính chia đa thức cho đa thức.

Công thức: $a^{m} : a^{n} = a^{m - n}$

Lời giải

Hãy liệt kê các hạng tử của đa thức B = 2x^3 - 3x^2 + x + 1 (ảnh 1)

Hãy liệt kê các hạng tử của đa thức B = 2x^3 - 3x^2 + x + 1 (ảnh 2)

Bài 7.28 trang 34 sách bài tập Toán 7: Khi làm phép chia (6x³ - 7x² - x + 2) : (2x - 1), bạn Quỳnh cho kết quả đa thức dư là 4x + 2.

a) Không làm phép chia, hãy cho biết bạn Quỳnh đúng hay sai, tại sao?

b) Tìm thương và dư trong phép chia đó.

Phương pháp giải

a) Chú ý bậc của đa thức dư.

b) Đặt phép tính chia 2 đa thức trên.

Lời giải

Quỳnh sai.

Bạn Quỳnh cho kết quả đa thức dư là 4x + 2, mà dư vẫn tiếp tục chia được cho 2x + 1.

Vậy bậc của đa thức dư, nếu khác 0, phải nhỏ hơn bậc của đa thức chia.

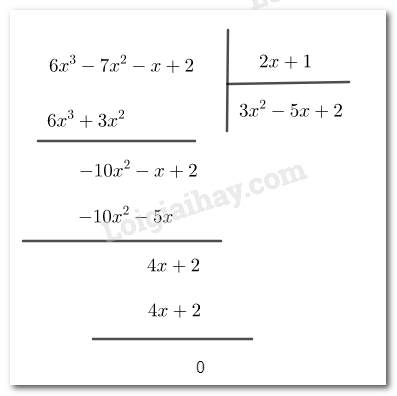
Thu gọn đa thức: P = 2x^3 - 5x^2 + 4x^3 + 4x + 9 + x (ảnh 1)

Thương là $3 x^{2} - 5 x + 2$

Dư là 0.

Bài 7.29 trang 34 sách bài tập Toán 7: Cho hai đa thức A = 3x⁴ + x³ + 6x - 5; B = x² + 1 . Tìm thương Q và dư R trong phép chia A cho B rồi kiểm nghiệm lại rằng A = BQ + R.

Phương pháp giải

Đặt phép tính chia A cho B để tìm thương Q và số dư R

Lời giải

Vậy chia A cho B ta được thương là $Q = 3 x^{2} + x - 3$ và số dư $R = 5 x - 2$ .

Bài 7.30 trang 34 sách bài tập Toán 7: Thực hiện các phép chia sau: a) ( 2x⁴ + x³ - 3x2 +5x - 2) : (x² - x + 1)

$a) (2 x^{4} + x^{3} - 3 x^{2} + 5 x - 2) : (x^{2} - x + 1)$

b) $(x^{4} - x^{3} - x^{2} + 3 x) : (x^{2} - 2 x + 3)$

Phương pháp giải

Đặt phép tính chia đa thức cho đa thức.

Công thức: $a^{m} : a^{n} = a^{m - n}$

Lời giải

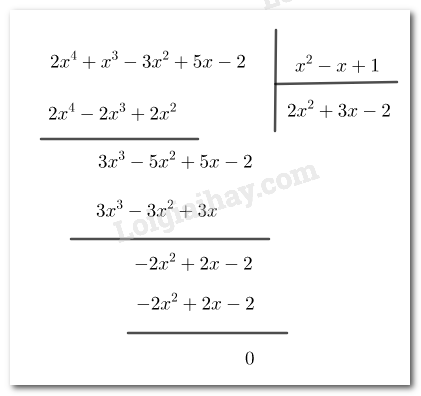
Xét đa thức P = - 3x^4 + 5x^2 - 2x + 1. Đó là một đa thức rút gọn (ảnh 1)

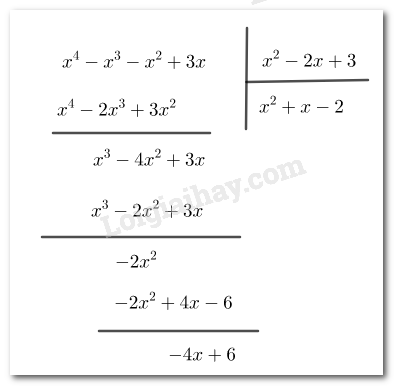
Xét đa thức P = - 3x^4 + 5x^2 - 2x + 1. Đó là một đa thức rút gọn (ảnh 2)

Bài 7.31 trang 34 sách bài tập Toán 7: Cho đa thức A(x) = 3x4 + 11x3 - 5x2 - 19x + 10 . Tìm đa thức H(x) sao cho A(x) = (3x2 +2x - 5). H(x)

Phương pháp giải

$\begin{array}{l} A (x) = (3 x^{2} + 2 x - 5) . H (x) . \\ \Rightarrow H (x) = A (x) : (3 x^{2} + 2 x - 5) \\ \Rightarrow H (x) = (3 x^{4} + 11 x^{3} - 5 x^{2} - 19 x + 10) : (3 x^{2} + 2 x - 5) \end{array}$

Đặt phép tính chia để tìm H(x).

Lời giải

Xét đa thức P = - 3x^4 + 5x^2 - 2x + 1. Đó là một đa thức rút gọn (ảnh 1)

Vậy $H (x) = x^{2} + 3 x - 2$ .

Bài 7.32 trang 34 sách bài tập Toán 7: Tìm số m sao cho đa thức P(x) = 2x³ - 3x² + x+ m chia hết cho đa thức x + 2.

Phương pháp giải

Để đa thức $P (x) = 2 x^{3} - 3 x^{2} + x + m$ chia hết cho đa thức x + 2 thì dư của phép chia bằng 0

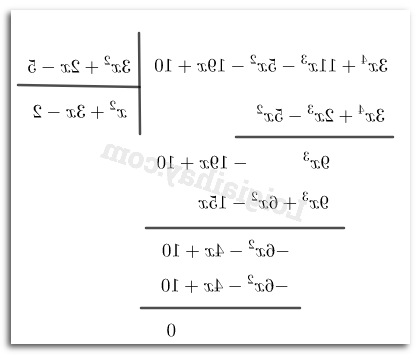
Thực hiện phép chia P(x) cho (x + 2)

Lời giải

Thực hiện phép chia P(x) cho (x + 2) ta được:

Xét đa thức P = - 3x^4 + 5x^2 - 2x + 1. Đó là một đa thức rút gọn (ảnh 1)

Để phép chia này là phép chia hết thì $m - 30 = 0 \Rightarrow m = 30$ .

Bài 7.33 trang 34 sách bài tập Toán 7: Cho đa thức P(x). Chứng minh rằng: a) Nếu P(x) chia hết cho x – a thì a là một nghiệm của đa thức P(x);

b) Nếu x = a là một nghiệm của đa thức P(x) thì P(x) chia hết cho x – a.

Phương pháp giải

a) $P (x) = (x - a) . Q (x) (1)$

Chứng minh P(a) = 0

b) $P (x) = (x - a) Q (x) + R (x)$

Chứng minh R(x) = 0 bằng phương pháp phản chứng.

Lời giải

Giả sử P(x) chia hết cho x – a. Gọi Q(x) là đa thức thương, ta có:

$P (x) = (x - a) . Q (x) (1)$

$\begin{array}{l} \Rightarrow P (a) = (a - a) . Q (a) \\ \Rightarrow P (a) = 0 \end{array}$

Vậy a là một nghiệm của P(x)

Ngược lại, cho a là một nghiệm của P(x). Giả sử chia P(x) cho x – a, ta được thươngg là Q(x) và dư là R(x), nghĩa là ta có: $P (x) = (x - a) Q (x) + R (x)$ (2)