Bài 1.8 trang 14 Toán 12 Tập 1 | Kết nối tri thức Giải Toán lớp 12

Bài 1.8 trang 14 Toán 12 Tập 1 | Kết nối tri thức Giải Toán lớp 12

Mai Hương Ngày: 14-03-2024 Lớp 12

175

Với giải Bài 1.8 trang 14 Toán 12 Tập 1 Kết nối tri thức chi tiết trong Bài 1: Tính đơn điệu và cực trị của hàm số giúp học sinh dễ dàng xem và so sánh lời giải, từ đó biết cách làm bài tập Toán 12. Mời các bạn đón xem:

Bài 1.8 trang 14 Toán 12 Tập 1 | Kết nối tri thức Giải Toán lớp 12

Bài 1.8 trang 14 SGK Toán 12 Tập 1: Cho hàm số $y = f (x) = | x |$ .

a) Tính các giới hạn $lim_{x \to 0^{+}} \frac{f (x) - f (0)}{x - 0}$ và $lim_{x \to 0^{-}} \frac{f (x) - f (0)}{x - 0}$ . Từ đó suy ra hàm số không có đạo hàm tại $x = 0$ .
b) Sử dụng định nghĩa, chứng minh hàm số có cực tiểu tại $x = 0$ . (Xem Hình 1.4)

Lời giải:

a) $lim_{x \to 0^{+}} \frac{f (x) - f (0)}{x - 0} = lim_{x \to 0^{+}} \frac{| x | - 0}{x - 0} = lim_{x \to 0^{+}} \frac{x}{x} = 1$

$lim_{x \to 0^{-}} \frac{f (x) - f (0)}{x - 0} = lim_{x \to 0^{-}} \frac{| x | - 0}{x - 0} = lim_{x \to 0^{-}} \frac{- x}{x} = - 1$

Vì $lim_{x \to 0^{+}} \frac{f (x) - f (0)}{x - 0} \neq lim_{x \to 0^{-}} \frac{f (x) - f (0)}{x - 0}$ nên hàm số không có đạo hàm tại $x = 0$ .

b) Đồ thị hàm số $y = f (x) = | x |$ :

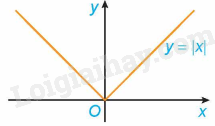
Ta có: $y = f (x) = | x | = {\begin{cases} - x k h i x \in (- \infty; 0) \\ x k h i x \in (0; + \infty) \end{cases}$

Hàm số $y = f (x) = | x |$ liên tục và xác định trên $(- \infty; + \infty)$

Với số $h > 0$ ta có: Với $x \in (- h; h) \subset (- \infty; + \infty)$ và $x \neq 0$ thì $y = f (x) = | x | > 0 = f (0)$

Do đó, hàm số $y = f (x) = | x |$ có cực tiểu là $x = 0$ .

Xem thêm các bài giải SGK Toán lớp 8 Kết nối tri thức hay, chi tiết khác:

Hoạt động 1 trang 6 SGK Toán 12 Tập 1: Quan sát đồ thị của hàm số $y = x^{2}$ (H.1.2)

Luyện tập 1 trang 6 SGK Toán 12 Tập 1: Hình 1.5 là đồ thị của hàm số $y = x^{3} - 3 x^{2} + 2$ . Hãy tìm các khoảng đồng biến, khoảng nghịch biến của hàm số.

Hoạt động 2 trang 6 SGK Toán 12 Tập 1: a) Xét dấu đạo hàm của hàm số trên các khoảng $(- \infty; - 1)$ , $(1; + \infty)$ .

Luyện tập 2 trang 7 SGK Toán 12 Tập 1: Tìm các khoảng đồng biến, khoảng nghịch biến của hàm số $y = - x^{2} + 2 x + 3$ .

Hoạt động 3 trang 7 SGK Toán 12 Tập 1: Cho hàm số $y = f (x) = x^{3} - 3 x^{2} + 2 x + 1$ .

Luyện tập 3 trang 9 SGK Toán 12 Tập 1: Tìm các khoảng đơn điệu của các hàm số sau:

Vận dụng 1 trang 9 SGK Toán 12 Tập 1: Giải bài toán trong tình huống mở đầu bằng cách thực hiện lần lượt các yêu cầu sau:

Hoạt động 4 trang 9 SGK Toán 12 Tập 1: Quan sát đồ thị của hàm số $y = x^{3} + 3 x^{2} - 4$ (H.1.7). Xét dấu đạo hàm của hàm số đã cho và hoàn thành các bảng sau vào vở:

Luyện tập 4 trang 10 SGK Toán 12 Tập 1: Hình 1.9 là đồ thị của hàm số $y = f (x)$ . Hãy tìm các cực trị của hàm số.

Hoạt động 5 trang 10 SGK Toán 12 Tập 1: Cho hàm số $y = \frac{1}{3} x^{3} - 3 x^{2} + 8 x + 1$ .

Câu hỏi trang 11 SGK Toán 12 Tập 1: Giải thích vì sao nếu f’(x) không đổi dấu qua $x_{0}$ thì $x_{0}$ không phải là điểm cực trị của hàm số f(x)?

Luyện tập 5 trang 12 SGK Toán 12 Tập 1: Tìm cực trị của các hàm số sau:

Vận dụng 2 trang 12 SGK Toán 12 Tập 1: Một vật được phóng thẳng đứng lên trên từ độ cao 2m với vận tốc ban đầu là 24,5m/s.

Bài 1.1 trang 13 SGK Toán 12 Tập 1: Tìm các khoảng đồng biến, khoảng nghịch biến của các hàm số có đồ thị như sau:

Bài 1.2 trang 13 SGK Toán 12 Tập 1: Xét sự đồng biến, nghịch biến của các hàm số sau:

Bài 1.3 trang 13 SGK Toán 12 Tập 1: Tìm các khoảng đơn điệu của các hàm số sau:

Bài 1.4 trang 13 SGK Toán 12 Tập 1: Xét chiều biến thiên của các hàm số sau:

Bài 1.5 trang 13 SGK Toán 12 Tập 1: Giả sử số dân của một thị trấn sau t năm kể từ năm 2000 được mô tả bởi hàm số $N (t) = \frac{25 t + 10}{t + 5}, t \geq 0$ , trong đó N(t) được tính bằng nghìn người.

Bài 1.6 trang 14 SGK Toán 12 Tập 1: Đồ thị của đạo hàm bậc nhất $y = f^{'} (x)$ của hàm số f(x) được cho trong Hình 1.13:

Bài 1.7 trang 14 SGK Toán 12 Tập 1: Tìm cực trị của các hàm số sau: a)

y = 2 x^{3} - 9 x^{2} + 12 x - 5

y = x^{4} - 4 x^{2} + 2

Bài 1.8 trang 14 SGK Toán 12 Tập 1: Cho hàm số

y = f (x) = | x |

Bài 1.9 trang 14 SGK Toán 12 Tập 1: Giả sử doanh số (tính bằng số sản phẩm) của một sản phẩm mới (trong vòng một số năm nhất định) tuân theo quy luật logistic được mô hình hóa bằng hàm số

Xem thêm các bài giải SGK Toán lớp 8 Kết nối tri thức hay, chi tiết khác:

Từ khóa :

Giải bài tập Toán 12

Đánh giá

0

0 đánh giá

Đánh giá

Bài viết cùng môn học

Toán Lớp 6

Toán 6 ( Kết nối tri thức): Luyện tập chung

Toán 6 ( Kết nối tri thức): Luyện tập chung Mai Hương

249

Toán Lớp 6

Bài 5.16 trang 109 Toán 6 Tập 1 | Kết nối tri thức Giải Toán lớp 6

Bài 5.16 trang 109 Toán 6 Tập 1 | Kết nối tri thức Giải Toán lớp 6 Mai Hương

286

Toán Lớp 6

Bài 5.15 trang 109 Toán 6 Tập 1 | Kết nối tri thức Giải Toán lớp 6

Bài 5.15 trang 109 Toán 6 Tập 1 | Kết nối tri thức Giải Toán lớp 6 Mai Hương

308

Toán Lớp 6

Bài 5.14 trang 109 Toán 6 Tập 1 | Kết nối tri thức Giải Toán lớp 6

Bài 5.14 trang 109 Toán 6 Tập 1 | Kết nối tri thức Giải Toán lớp 6 Mai Hương

258

Tìm kiếm

Bài Viết Xem Nhiều

Bài Viết Cùng Lớp

CÔNG TY TNHH ĐẦU TƯ VÀ DỊCH VỤ GIÁO DỤC VIETJACK

- Người đại diện: Nguyễn Thanh Tuyền

- Số giấy chứng nhận đăng ký kinh doanh: 0108307822, ngày cấp: 04/06/2018, nơi cấp: Sở Kế hoạch và Đầu tư thành phố Hà Nội.

2021 © All Rights Reserved.