SBT Toán 11 trang 10 Tập 1 (Cánh Diều)

Ngọc Nguyễn Ngày: 12-11-2023 Lớp 11

238

Với Giải trang 10 Tập 1 SBT Toán lớp 11 trong Bài 1: Góc lượng giác. Giá trị lượng giác của góc lượng giác Sách bài tập Toán lớp 11 Cánh Diều hay nhất, chi tiết sẽ giúp học sinh dễ dàng làm bài tập trong SBT Toán lớp 11.

Nội dung bài viết

Bài 1 trang 10 SBT Toán 11 Tập 1
Bài 2 trang 10 SBT Toán 11 Tập 1
Bài 3 trang 10 SBT Toán 11 Tập 1
Bài 4 trang 10 SBT Toán 11 Tập 1
Bài 5 trang 10 SBT Toán 11 Tập 1
Bài 6 trang 10 SBT Toán 11 Tập 1

SBT Toán 11 trang 10 Tập 1 (Cánh Diều)

Bài 1 trang 10 SBT Toán 11 Tập 1: Trên đường tròn lượng giác lấy điểm M sao cho (OA, OM) = 40°. Gọi M' đối xứng với M qua gốc toạ độ. Khi đó số đo của góc lượng giác (OA, OM') bằng:

A. 40°+ k360°.

B. 140°+ k360°.

C. 220°+ k360°.

D. 50° + k360°.

Lời giải:

Đáp án đúng là: C

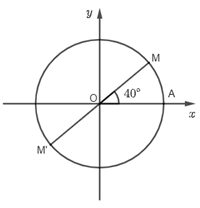
SBT Toán 11 (Cánh diều) Bài 1: Góc lượng giác. Giá trị lượng giác của góc lượng giác (ảnh 1)

Vì M, M' đối xứng nhau qua gốc tọa độ O nên M, O, M' thẳng hàng.

Ta có:

(OA, OM') = (OA, OM) + (OM, OM') + k360°

= 40° + 180° + k360° = 220° + k360°.

Bài 2 trang 10 SBT Toán 11 Tập 1: Cho $\cos α = - \frac{2}{5}$ với $\frac{π}{2} < α < π$ . Khi đó, tan α bằng:

A. $\frac{\sqrt{21}}{5}$ .

B. $- \frac{\sqrt{21}}{2}$ .

C. $\frac{\sqrt{21}}{2}$ .

D. $- \frac{\sqrt{21}}{5}$ .

Lời giải:

Đáp án đúng là: B

Vì $\frac{π}{2} < α < π$ nên tan α < 0.

Do đó, từ $1 + \tan^{2} α = \frac{1}{\cos^{2} α}$ , ta suy ra

$\tan α = - \sqrt{\frac{1}{\cos^{2} α} - 1} = - \sqrt{\frac{1}{{(- \frac{2}{5})}^{2}} - 1} = - \frac{\sqrt{21}}{2}$ .

Bài 3 trang 10 SBT Toán 11 Tập 1: Cho tan α + cot α = 2. Khi đó, tan²α + cot²α bằng:

A. 8.

B. 4.

C. 16.

D. 2.

Lời giải:

Đáp án đúng là: D

Ta có tan α + cot α = 2

Suy ra (tan α + cot α)² = 2² = 4.

Mà (tan α + cot α)² = tan² α + 2tan α . cot α + cot² α

= tan² α + 2 . 1 + cot² α = tan² α + cot² α + 2 = 4.

Do đó, tan²α + cot²α = 4 – 2 = 2.

Bài 4 trang 10 SBT Toán 11 Tập 1: Kết quả thu gọn của biểu thức

$A = \sin (π + x) + \cos (\frac{π}{2} - x) + \cot (2 π - x) + \tan (\frac{3 π}{2} + x)$ là:

A. – 2cot x.

B. 2tan x.

C. 2sin x.

D. – 2sin x.

Lời giải:

Đáp án đúng là: A

$A = \sin (π + x) + \cos (\frac{π}{2} - x) + \cot (2 π - x) + \tan (\frac{3 π}{2} + x)$

$= - \sin x + \sin x + \cot (π + π - x) + \tan (π + \frac{π}{2} + x)$

$= \cot (π - x) + \tan (\frac{π}{2} + x)$

$= \cot (- x) + \tan (π + x - \frac{π}{2})$

Kết quả thu gọn của biểu thức A = sin (π + x) + cos(π/2 - x) + cot(2π - x) + tan(3π/2 + x)

$= - \cot x - \tan (\frac{π}{2} - x)$

$= - \cot x - \cot x = - 2 \cot x$

Bài 5 trang 10 SBT Toán 11 Tập 1: Cho tan α = 2. Khi đó giá trị của biểu thức $A = \frac{\sin^{2} α - 2 \sin α . \cos α}{\cos^{2} α + 3 \sin^{2} α}$ bằng:

A. 4.

B. 0.

C. 1.

D. 2.

Lời giải:

Đáp án đúng là: B

Vì tan α = 2 xác định nên cos α ≠ 0, hay cos² α ≠ 0, do đó chia cả tử và mẫu của A cho cos² α ta được:

$A = \frac{\frac{\sin^{2} α}{\cos^{2} α} - \frac{2 \sin α . \cos α}{\cos^{2} α}}{\frac{\cos^{2} α}{\cos^{2} α} + \frac{3 \sin^{2} α}{\cos^{2} α}} = \frac{\tan^{2} α - 2 \tan α}{1 + 3 \tan^{2} α} = \frac{2^{2} - 2.2}{1 + {3.2}^{2}} = 0$ .

Bài 6 trang 10 SBT Toán 11 Tập 1: Cho lục giác đều ABCDEF nội tiếp trong đường tròn lượng giác (thứ tự đi từ A đến các đỉnh theo chiều ngược chiều kim đồng hồ). Tính số đo của các góc lượng giác (OA, OB), (OA, OC), (OA, OD), (OA, OE), (OA, OF).

Lời giải:

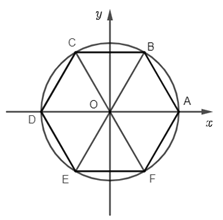
SBT Toán 11 (Cánh diều) Bài 1: Góc lượng giác. Giá trị lượng giác của góc lượng giác (ảnh 2)