Top 1000 câu hỏi thường gặp môn Toán có đáp án (phần 25) hay nhất được biên soạn và chọn lọc giúp bạn ôn luyện và đạt kết quả cao trong bài thi môn Toán.
Cho a, b, c đôi một khác nhau thỏa mãn (a + b + c)2 = a2 + b2 + c2.
Câu 23: Cho a, b, c đôi một khác nhau thỏa mãn (a + b + c)2 = a2 + b2 + c2.
Tính .
Lời giải:
Ta có (a + b + c)2 = a2 + b2 + c2
⇔ a2 + b2 + c2 + 2ab + 2bc + 2ac = a2 + b2 + c2
⇔ 2ab + 2bc + 2ac = 0
⇔ ab + bc + ac = 0
⇔
Thay vào biểu thức P ta có
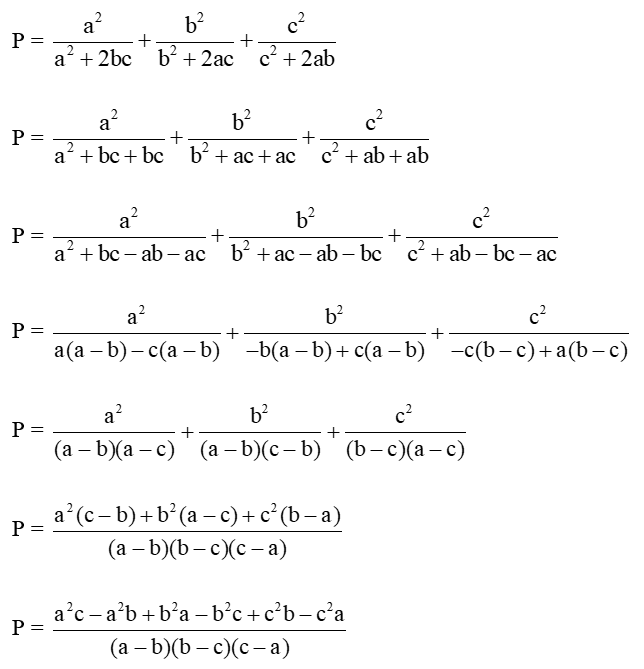
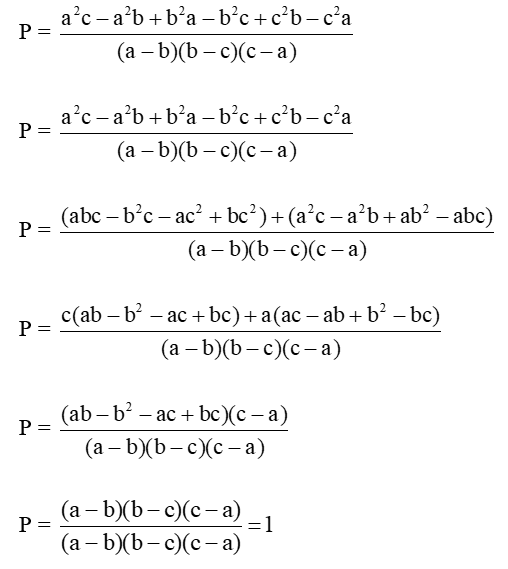
Vậy P = 1.
Bài viết cùng bài học:




